A Complete Guide to Capital Asset Pricing Model (CAPM)

There is always some level of risk when it comes to investments no matter how much you diversify them. To compensate for that risk, investors look for a rate of return. Investors use the capital asset pricing model (CAPM) to calculate investment risk and what return on investment they can expect.

Capital Asset Pricing Model

The Capital Asset Pricing Model (CAPM) calculates an investment’s expected return based on its systematic risk. The CAPM is used to compute the cost of equity, which is defined as the needed rate of return for equity investors. The CAPM, which ties the predicted return on a security to its sensitivity to the wider market, is the most prevalent method for calculating the cost of equity.

What is the Capital Asset Pricing Model (CAPM)?

The Capital Asset Pricing Model (CAPM) is a mathematical model that describes the link between a security’s expected return and risk. It demonstrates that a security’s expected return is equal to the risk-free return plus a risk premium based on the security’s beta. CAPM is a specialized model used in business finance to analyze the relationship between expected dividends and the risk of investing in a specific company.

Importance of CAPM

Every investment entails some level of risk. Even equity carries the risk of a discrepancy between the actual and expected returns. The cost of equity is the discount rate applied to expected equity cash flows to help an investor evaluate the price they are willing to pay for those cash flows. Investors, by nature, are risk-averse and will only accept a risk if they can predict the expected return on investment. Investors can use CAPM to compute and estimate the required return on investment based on a risk assessment.

How does CAPM help investors?

When determining the fair value of a stock, investors employ CAPM. As a result, when the number of risk changes or other market circumstances makes an investment riskier, they’ll use the formula to help re-price and anticipate potential returns. When it comes to investing, the larger the risk, the higher the expected return. The capital asset pricing model (CAPM) attempts to calculate how much you can anticipate earning based on the level of risk. When making investment decisions, the model is frequently utilized in conjunction with fundamental analysis, technical analysis, and other methods of sizing up assets.

• Assumption of a diversified portfolio – Risk aversion is a common trait among investors. They want to put their money into a low-risk portfolio. As a result, investors expect a bigger return if a portfolio has a higher risk. Investors examine only a one-period horizon while making investment decisions, rather than many period horizons. In the financial market, transaction costs are believed to be low, and investors can buy and sell assets in unlimited quantities at a risk-free rate of return.
• Convenient and simple – The CAPM is a straightforward formula that can be easily stress-tested to generate a range of possible outcomes and ensure that the needed rates of return are met. In many cases, the CAPM produces a more helpful result than either the DDM or the WACC models.
• Systematic risks can alter this calculation significantly – In CAPM, the beta factor considers any systematic risks associated with an investment. DDM, or dividend discount model, disregards the effects of such risks on returns. Since market risk can;t be predicted, no investor can mitigate its effects in their entirety.

Pros and cons of CAPM

CAPM is a financial theory that proposes a linear link between an investment’s necessary return and risk. It offers both advantages and disadvantages when applied in practice:

Pros of CAPM

The CAPM has a number of advantages over alternative methods for computing needed returns, which is why it has been so popular for over 40 years:

• It only examines systematic risk, reflecting the reality that most investors have well-diversified portfolios with little or no unsystematic risk.
• It is a theoretically generated link between necessary return and systematic risk that has been empirically researched and tested numerous times.
• It is often regarded as a far superior technique of estimating the cost of equity to the dividend growth model (DGM). It clearly analyses a company’s level of systematic risk compared to the stock market as a whole.

Cons of CAPM

A fair examination of this significant theoretical model should include a discussion of the CAPM’s shortcomings and limitations.

• The risk-free rate of return, the return on the market, the equity risk premium (ERP), and the equity beta must all have values allocated to them to employ the CAPM.
• The yield on short-term government debt, which is used to replace the risk-free rate of return, is not constant and fluctuates with changing economic conditions. This volatility can be smoothed out using a short-term average value.
• It’s more challenging to calculate the equity risk premium (ERP). The average capital gain plus the average dividend yield equals the return on a stock market.

Calculation of CAPM

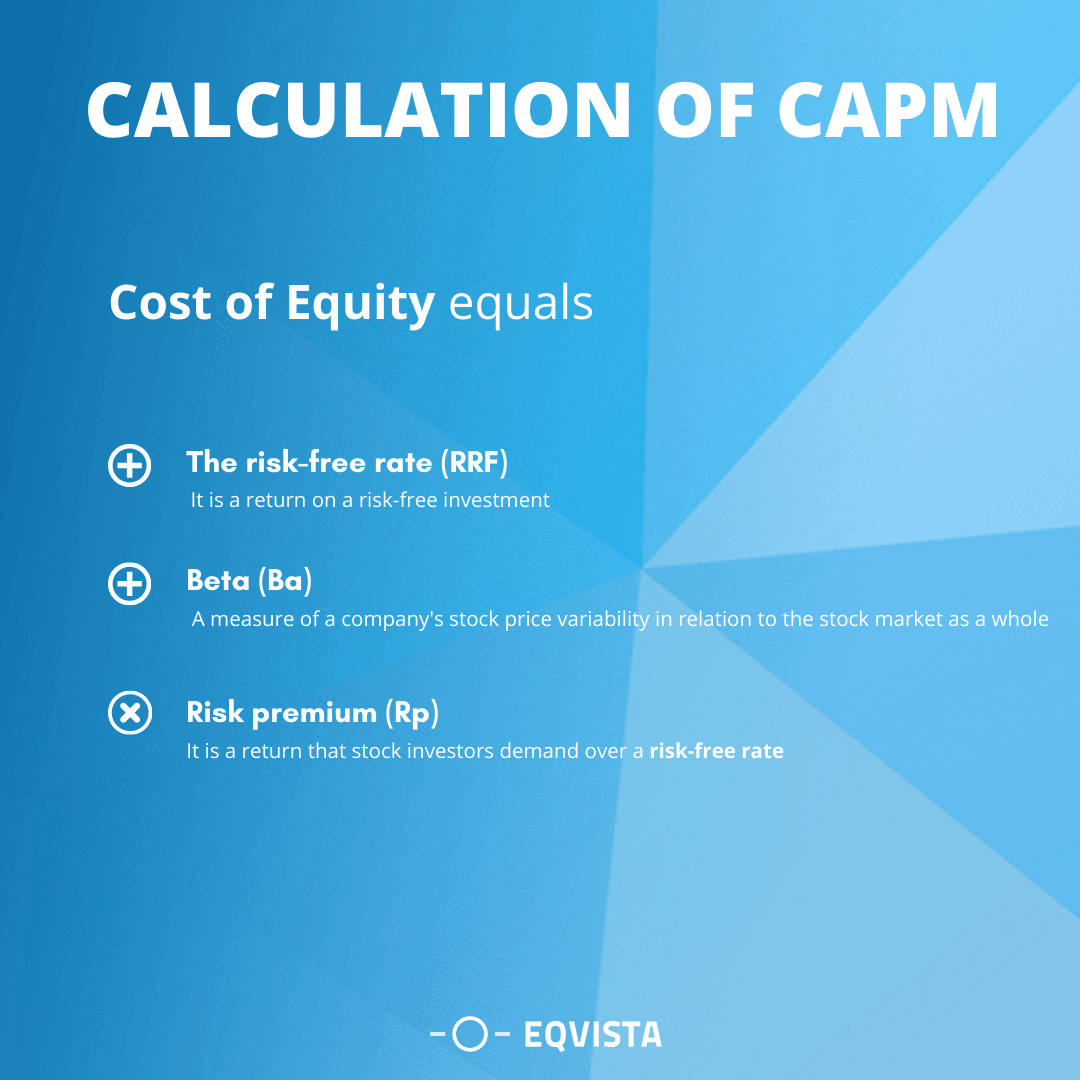
The rate of return expected by shareholders is known as the cost of equity (Ke). The formula can be used to compute it.

Where:

• The rate of return expected by shareholders (Ke) is the cost of equity (Ke).
• The risk-free rate (rrf) is the return on a risk-free investment.
• The return that stock investors demand over a risk-free rate is known as the risk premium (Rp).
• Beta (Ba) = A measure of a company’s stock price variability in relation to the stock market as a whole.

Formula of CAPM

The capital asset pricing version offers a formulation that calculates the anticipated return on a safety primarily based totally on its hazard stage. The capital asset pricing version system is the chance of loose fee plus beta instances, the distinction of the go back available in the marketplace and the threat of loose price.

• Expected return – The stock expected dividends and capital appreciation are projected by the CAPM formula. It is used to calculate the discount on the stock’s dividend over a long holding period. There might be a risk if the stock is relatively valued to the discounted value of cash flow.
• Risk-free rate – It is the least rate of return that an investor can expect from a risk-free investment. Government bonds are regarded as risk-free investments. There should be no default risk or reinvestment risk for a security to be considered risk-free. The possibility of a company or an individual defaulting on their debt obligations is known as default risk. The danger that an investor faces when the bond yield falls is known as reinvestment risk. As a result of this predicament, investors must reinvest their future income, yield, or final return of principal in lower-yielding securities. As a result, not all government securities are risk-free.
• Beta – There are two types of risk: systematic risk and unsystematic risk. The risk that can be diversified is known as unsystematic risk. This risk emerges as a result of internal causes in the organization. Workers have gone on strike, consumer agreements have not been met, the regulatory system is at odds with government policy, and so on. A risk that cannot be diversified is known as systematic risk. It is caused by external variables that have an impact on a company. In nature, it is uncontrollable. For example, interest rates abruptly rise, fluctuations in a security’s trading price have an impact on the entire market, and so on. A beta can be used to quantify this risk.
• Market Risk Premium – Stock market return minus the risk-free rate of return is the formula used to calculate it. It is the additional return that an investor expects over the risk-free rate of return to compensate for the risk of volatility that comes with investing in the stock market. For example, if the investor receives a 20% return and the risk-free rate is 7%, the risk premium is 13%.

Calculation Example of CAPM

Let’s use the Capital Asset Pricing Model (CAPM) formula to calculate the expected return on a stock. Assume you have the following information about a stock:

• Its operations are based in the United States, and it trades on the New York Stock Exchange.
• The current yield on a 10-year Treasury bill in the United States is 2.5 percent.
• The average excess annual return on US stocks in the past has been 7.5 percent.
• The stock’s beta is 1.25. (meaning its average return is 1.25x as volatile as the S&P 500 over the last 2 years)

Using the CAPM formula, what is the expected return on the security?

Let’s take a look at the answer using the formula from the previous section of the article:

• Risk Free Rate + [Beta x Market Return Premium] = Expected Return’]
• Return on investment = 2.5 percent plus [1.25 x 7.5 percent]
• 11.9 percent will be expected return

Drawbacks of capital assets pricing model

The notion of a single-period time horizon is incompatible with investment appraisal’s multi-period character. While CAPM variables can be considered to be constant throughout time, experience shows that this is not the case in the real world. Despite its frequent use, the CAPM calculation is a false indicator of the potential rate of return. The CAPM’s fundamental assumptions are impractical and have little application in the real world of investing.

CAPM and its frontier

Using the CAPM to construct a portfolio is designed to assist an investor in risk management. If a portfolio’s return relative to risk could be perfectly optimized using the CAPM, it would exist on a curve known as the efficient frontier, as depicted in the graph below.

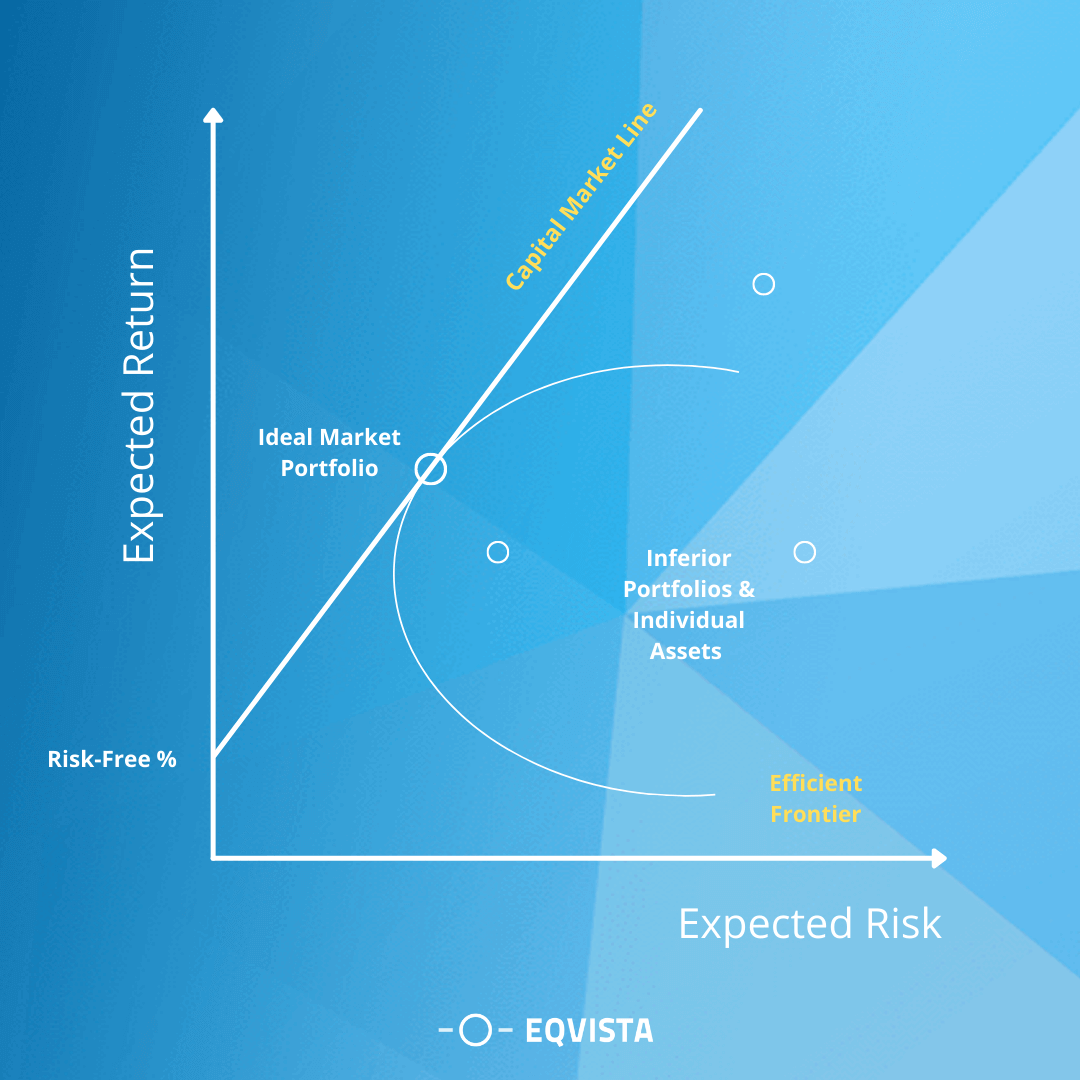
The graph illustrates how higher expected profits (y-axis) necessitate higher expected risk (x-axis). According to Modern Portfolio Theory, a portfolio’s expected return increases as risk increases, starting with the risk-free rate. Any portfolio that fits on the Capital Market Line (CML) is better than any portfolio that fits the right of that line, but at some point on the CML, a theoretical portfolio with the best return for the amount of risk taken can be formed.

Although the CML and efficient frontier are difficult to define, they demonstrate a crucial notion for investors: more yield comes at the expense of increased risk.

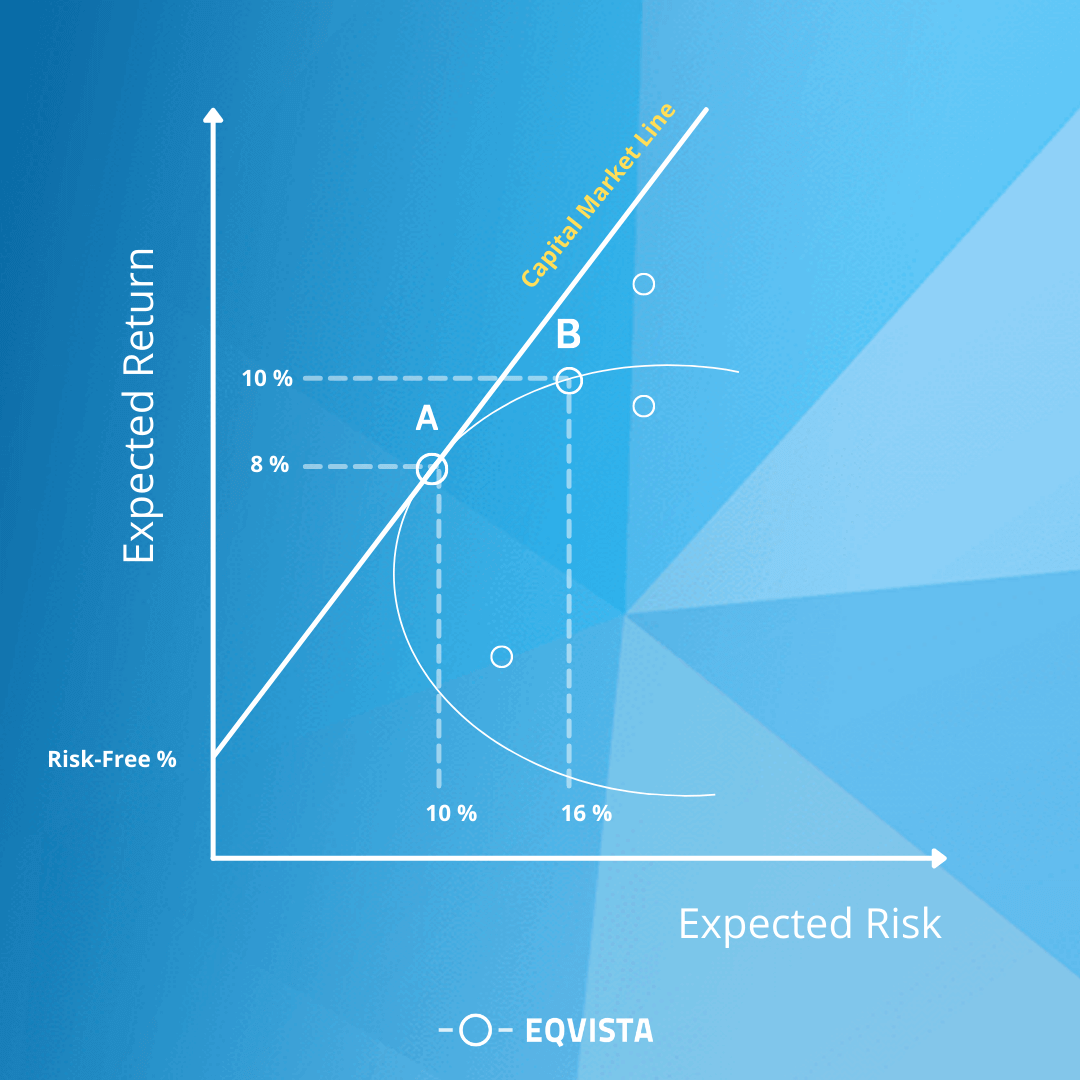
Two portfolios that have been built to fit along the efficient frontier are shown in the chart below. Portfolio A is predicted to return 8% per year and has a risk level of 10% standard deviation. Portfolio B is expected to return 10% per year but has a standard deviation of 16%. Portfolio B’s risk increased quicker than its predicted returns.

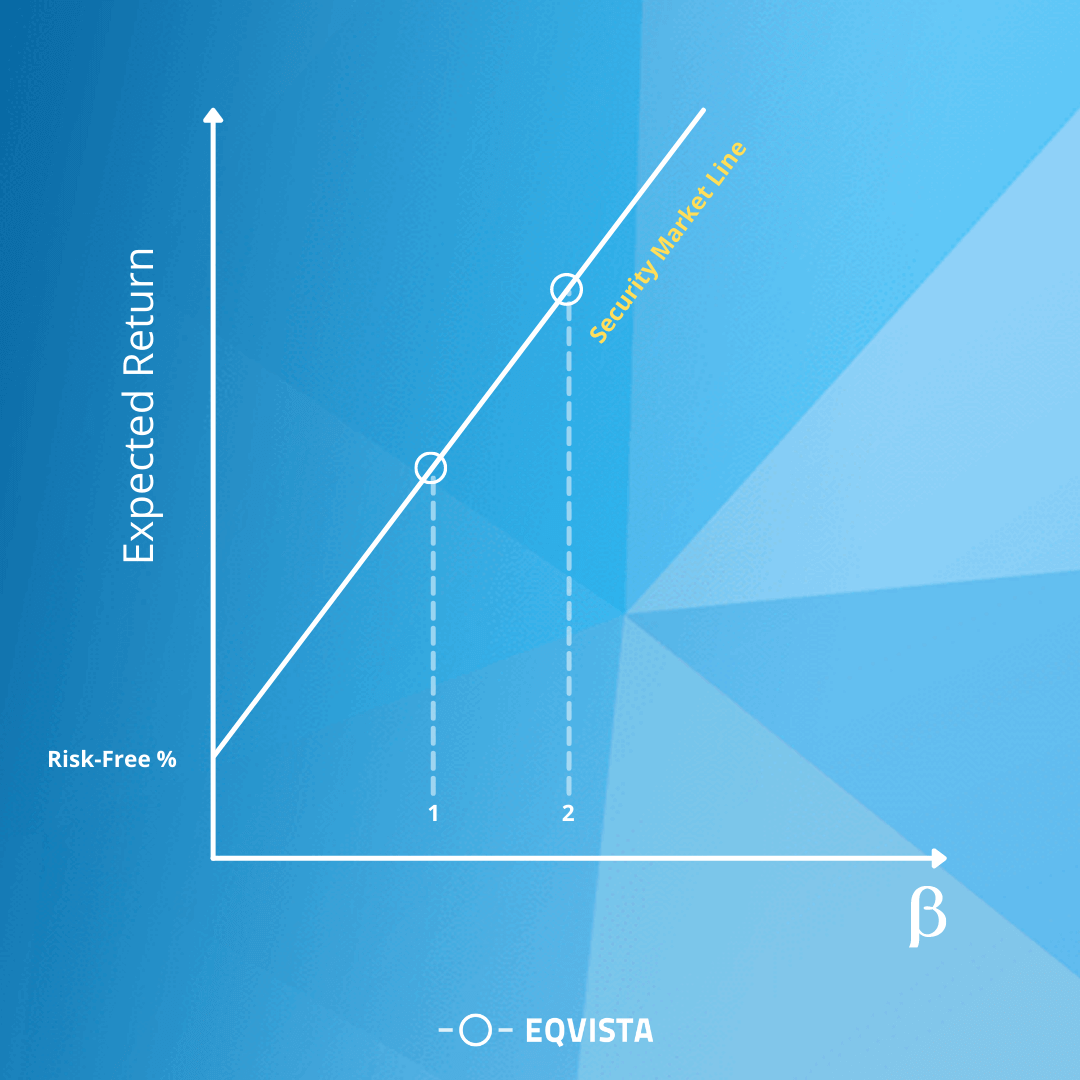
The CAPM and SML establish a link between the beta of a stock and its predicted risk. Although a higher beta implies greater risk, a portfolio of high beta equities could exist somewhere on the CML where the trade-off is acceptable, if not optimal.

These two models’ usefulness is harmed by assumptions about beta and market participants that aren’t true in real markets. For example, beta does not take into account the relative riskiness of a stock that is more volatile than the market and experiences a high frequency of downside shocks vs a stock with an equivalent high beta that does not experience the same price moves to the downside.

Manage your equity stock with Eqvista!

If you’re looking for an expert to manage your equity stock. All you have to do now is contact us to start talking about creating a plan, learning everything there is to know about managing your stock. Eqvista is here to assist you, and don’t hesitate to get in touch with us, fill the contact form now.