How To Value Carried Interest Valuations for Private Equity?

Carried interest, often termed “carry,” is essentially a share of the profits private equity fund managers earn from investments. This mechanism incentivizes fund managers to excel and aligns their interests with those of the fund’s investors. Historically, carry has been a substantial part of a fund manager’s income. 

Understanding how to value carried interest in PE is crucial for stakeholders, including LPs assessing investment opportunities and GPs managing fund performance. This article is everything you need to learn about carried interest valuation methods in private equity.

What is carried interest?

Carried interest in private equity refers to the share of profits that general partners receive as compensation for managing the investment fund. Essentially, it’s a share of the fund’s profits, awarded to the managers and meets certain performance benchmarks, often referred to as a “hurdle rate”. 

In the US , the treatment of carried interest has been a topic of debate, especially regarding how it should be taxed. Currently, this is taxed at capital gains rates,leading to discussions about whether this is fair or there is a need for a change in tax policy.

How does carried interest work in private equity?

When a private equity fund invests in companies, the goal is to improve those companies’ value and sell them at a profit. If the fund does well and surpasses the predefined hurdle rate, fund managers receive a portion of the profits, typically around 20%.

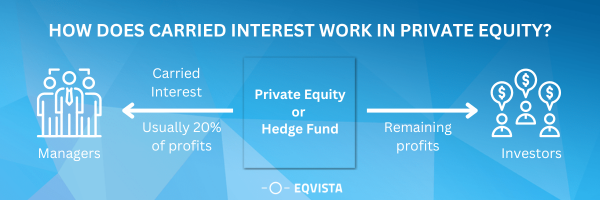
By having their compensation tied directly to the fund’s performance, managers are more likely to make decisions that benefit the fund.

The carried interest valuation can be complex. It depends on various factors, including the fund’s overall performance, the specific terms of its agreement regarding and the timing of investments and exit. The carried 

How to value carried interest in Private Equity?

Valuing carried interest in private equity is a complex process requiring a deep understanding of the fund’s operational mechanics and the broader market environment.

• Fund Agreement Provisions: The specifics of the fund agreement significantly influence valuation, particularly terms related to profit allocations, distribution provisions, and hurdle rates.
• Discount Rates: The choice of discount rate is vital, reflecting the risk profile of the investment. Market data and industry studies help in setting appropriate rates.
• Expected Returns: Projections of fund performance based on historical data and market conditions are essential for accurate valuation.

Carried Interest valuation methods

The following carried interest valuation methods offer a structured approach to quantifying the potential value. It also balances the need for precision with the inherent uncertainties of investment performance.

Discounted Cash Flow (DCF) Analysis

DCF is a common approach for valuing carried interest, particularly effective for modeling various assumptions, return structures, and incorporating the nuances of management fees, allocation waterfalls, and return assumptions. The method discounts expected future cash flows to their present value, considering risk factors and the time value of money​​.

Example for DCF Analysis:

A private equity fund has a 20% carried interest, meaning the general partner (GP) receives 20% of the profits after investors receive their preferred return (e.g., 8%).The fund currently has $100 million in committed capital and is expected to invest all capital within 2 years.

The GP expects the fund to achieve a 15% annual return on invested capital (ROIC) over a 5-year investment period, followed by a 2-year liquidation period. The discount rate for this risky asset class is estimated at 12%.

Steps:

• Project cash inflows:
  • Calculate the expected profit by multiplying ROIC by invested capital and investment period: $100 million * 15% * 5 years = $75 million.
  • Apply the carried interest percentage to get the GP’s share: $75 million * 20% = $15 million.
  • Assume equal annual distributions over the liquidation period: $15 million / 2 years = $7.5 million per year.
• Discount cash flows:
  • Year 1: $7.5 million / (1 + 12%) = $6.73 million.
  • Year 2: $7.5 million / (1 + 12%)^2 = $6.09 million.
• Calculate present value:
  • Add the discounted cash flows: $6.73 million + $6.09 million = $12.82 million.

Therefore, the estimated value of the carried interest using DCF is $12.82 million.

Option Pricing Methods: 

These methods, including the Black-Scholes Model and Lattice Models, are useful, especially in the early stages of a fund when there is a lack of detailed forecasted cash flow data for the fund or its underlying investments. They rely on market data to estimate the value of carried interest as a call option on the share of the fund’s profits​​.

Example For Option Pricing Method:

Imagine a GP valuing a 20% carried interest in a private equity fund with a 5-year investment period and a 2% hurdle rate. The fund has raised $100 million and plans to invest it over the next year. He believes the fund can achieve an average 20% annual return, but the market is volatile, with a 30% standard deviation. The current risk-free rate is 3%.

Here’s how the option pricing method can estimate the carried interest’s value:

• Underlying Asset: The fund’s net asset value (NAV) is the underlying asset. Since its early stage, the NAV is simply the committed capital, which is $100 million.
• Exercise Price: This hurdle rate multiplied by the committed capital is $2 million ($100 million * 2%).
• Option: The carried interest acts like a call option on the NAV. The holder only gets paid if the NAV exceeds the hurdle rate.
• Model: The Black-Scholes model is commonly used for call options. It requires the following inputs:
  • Spot price (S): $100 million (NAV)
  • Strike price (X): $2 million (hurdle rate)
  • Time to expiration (T): 5 years
  • Volatility (σ): 30%
  • Risk-free rate (r): 3%
• Calculation: Plug these values into the Black-Scholes model to get the option value (carried interest value). In this example, it’s approximately $20 million.
• Interpretation: Based on these assumptions, the 20% carried interest is worth around $20 million. This represents the potential future value based on the fund’s expected performance.

Monte Carlo Simulation: 

This method simulates various possible outcomes for a fund’s performance based on input variables to estimate the value of carried interest. It’s particularly beneficial for modeling a wide range of future states but may be complex to adjust for specific provisions in fund agreements​​.

Example For Monte Carlo Simulation Method:

Scenario:

• Private equity fund with a 2/20 waterfall structure (2% management fee, 20% carried interest)
• Current fund size: $100 million
• Holding period: 5 years
• Exit multiples (enterprise value / invested capital) range from 1.5x to 3x (uniform distribution)
• Discount rate: 10%
• Number of iterations: 50 (reduced for simplicity)

Steps:

• Define Key Variables:
  • Investment size per period: $100 million / 5 years = $20 million
  • Management fee: $2 million/year
  • Carried interest hurdle: $100 million + ($2 million/year * 5 years) = $110 million
  • Waterfall parameters: 2% management fee, 20% carried interest on profits above the hurdle.
• Build the Simulation Model:
  • Use a spreadsheet or dedicated software and set up 50 iterations to represent different scenarios.

• Simulate Each Iteration:
  • Randomly draw an exit multiple from the defined range (1.5x to 3x).
  • Calculate each iteration’s enterprise value (EV): EV = Investment size * Exit multiple.
  • Calculate the total fund proceeds: Proceeds = EV – Management fees.
  • Calculate the carried interest amount:
    1. If Proceeds <= Hurdle: Carried interest = $0.
    2. If Proceeds > Hurdle: Carried interest = 0.2 * (Proceeds – Hurdle).
  • Discount the carried interest amount back to the present value using the discount rate.
• Analyze the Results:
  • Calculate the average present value of carried interest across all iterations. This represents the expected value of the carried interest.
  • Analyze the distribution of present values to understand the potential range of outcomes.
  • Calculate additional statistics like standard deviation and percentiles (but note their reduced reliability with 50 iterations).

Example:

Iteration	Exit Multiple	EV	Proceeds	Carried Interest	PV Carried Interest
1	2.5	$250 million	$238 million	$25.6 million	$19.6 million
2	1.7	$170 million	$158 million	$0	$0
...	...	...	...	...	...
50	2.8	$280 million	$268 million	$31.6 million	$23.9 million

• Average Present Value of Carried Interest:
  • Based on the 50 iterations, the average present value of carried interest in this example is approximately $15 million.

Present Value Determination: 

This approach calculates the present value of future cash flow or carried interest distributions expected over the fund’s life. It often uses an option-pricing framework or a discounted cash flow methodology to estimate this present value​​.

Example For Present Value Determination:

In private equity or hedge funds, carried interest represents a share of profits distributed to the investment manager or general partner. Determining the present value  involves applying the Discounted Cash Flow (DCF) analysis, a method used to estimate the current value of future cash flows. Let’s consider a simple example to illustrate this concept.

• Assumptions:
  • Carried Interest Percentage: 15%
  • Investment Horizon: 3 years
  • Projected Annual Cash Flow: $500,000
  • Discount Rate: 10%
• Present Value Formula:
  • The present value (PV) of carried interest over n years is calculated using the DCF formula:
  • PV = CF₁/(1+r)¹ + CF₂/(1+r)² +… + CF_n/(1+r)ⁿ

Where:

PV is the present value of carried interest.

CF_i is the cash flow in the year i.

R is the discount rate.

For our example:

PV_i = $500,000/(1 + 0.10)ⁱ

Calculating for each year (i=1 to 3):

• PV₁ = $500,000/1.10 ≈ $454,545.45
• PV₂ = $500,000/1.10² ≈ $454,545.45
• PV₃ = $500,000/1.10³ ≈ $375,657.89

Summing these present values:

PV = $454,545.45 + $454,545.45 + $375,657.89 ≈ $1,243,426.48

Therefore, the present value of the carried interest over the 3-year investment horizon is approximately $1,243,426.48.

Probability Weighted Expected Returns Approach (PWERM): 

This approach assesses a fund’s potential value during its early to mid-stages by predicting future  distributions under multiple scenarios—upside, base, and downside. It then discounts these projections to their present value to account for risk. This method incorporates a discount rate reflecting the forecasted distributions’ riskiness and a marketability discount due to the illiquid nature of carried interests.

Example For Probability Weighted Expected Returns Approach:

Let’s consider a venture capital fund that has invested in several startups. The fund manager expects to receive a carried interest of 20% on profits above a certain hurdle rate, say 8% per annum, after the return of the initial investment to the limited partners.

• Scenario 1: Bullish Outcome
  • Probability: 30%
  • Expected Return: 30%

• Scenario 2: Moderate Outcome
  • Probability: 50%
  • Expected Return: 15%
• Scenario 3: Bearish Outcome

• Probability: 20%
• Expected Return: 5%

To calculate the probability-weighted expected return for the fund, you multiply the probability of each scenario by its expected return and sum them up:

• Expected Return=(0.30×0.30)+(0.50×0.15)+(0.20×0.05)
• Expected Return=0.09+0.075+0.01=0.175

So, the expected return for the fund is 17.5%.

The carried interest is calculated based on the excess return above the hurdle rate. Let’s assume the fund size is $100 million.

• If the fund returns 17.5% annually, the total fund value at the end of the investment period would be $117.5 million.
• The hurdle rate is 8%, so the hurdle amount would be $8 million.
• The profits above the hurdle would be $117.5 million – $100 million = $17.5 million
• The carried interest would be 20% of the profits above the hurdle, which is $ 3.5 million.

This $3.5 million represents the compensation to the fund managers based on the probability-weighted expected returns approach.

Current Liquidation Scenario: 

The Current Liquidation Approach is used when carried interest is profitable post-catch-up or as the fund nears its lifecycle. It estimates the value of simulating a scenario where the fund is liquidated at the valuation date, relying on the current value of underlying investments. This method calculates expected carry distributions, assuming an immediate fund liquidation to determine the carried interest’s present worth based on existing investment values.

Current Liquidation Scenario Example:

Scenario:

• A private equity fund has $100 million in total investments.
• Investors initially contributed $80 million to the fund.
• The carried interest rate is 20%.
• The current market value of all investments is $120 million.

Steps:

1. Profit after repaying investors: $120 million (current value) – $80 million (initial investment) = $40 million.
2. Carried interest percentage: 20%
3. Estimated carried interest value: $40 million (profit) x 20% (carried interest rate) = $8 million

Therefore, based on the current liquidation approach, the estimated value of the carried interest is $8 million.

Factors influencing the valuation of Carried Interest in private equity

Carried interest valuation in private equity is a process influenced by various factors, each playing a crucial role in determining the fair market value. These factors include:

• Expected Timing of Distributions: The anticipated timing for distributions impacts valuation significantly. Carried interest benefits from future fund performances and distributions, making the timing a critical variable for valuation​​.
• Expected Volatility: The variability or uncertainty of fund returns affects carried interest value. Higher expected volatility in investment returns can increase the potential upside, thus impacting the valuation methods​​.
• Rights and Preferences Detailed in the Fund Agreement: The specific terms outlined in fund agreements, such as profit allocation, distribution provisions, and hurdle rates, are vital for understanding the valuation. These terms dictate the share of profits fund managers are entitled to and under what conditions, influencing the valuation​​​​.
• Selection of Valuation Methodology: The chosen carried interest valuation methods, whether a Discounted Cash Flow (DCF) analysis, option pricing methods, or Monte Carlo simulation, shape the valuation outcome. 
• Discount Rate: The rate used to discount future cash flows back to their present value is a significant determinant of carried interest valuation. This rate reflects the risk profile of the investment, where higher rates are used for higher-risk investments, affecting the present value calculation​​​​.
• Fund’s Life Cycle: The stage of the fund’s life cycle, whether early stage, later stage, or somewhere in between, influences expected returns and, consequently, the carried interest valuation. This factor ties closely with the fund’s anticipated timing of distributions and the volatility of its investments​​.

Get Accurate Valuation From Eqvista!

An accurate valuation ensures fair compensation based on fund performance, upholds compliance with regulatory standards and facilitates strategic financial planning. The precision of carried interest valuations is significant in private equity, where the stakes are high, and the outcomes significantly impact numerous stakeholders.

In this context, Eqvista is a pivotal resource for private equity firms and stakeholders seeking to navigate the intricacies of valuations. Offering a comprehensive suite of equity management solutions, we help demystify the valuation process with our advanced software and expert services. 

From cap table management to sophisticated valuation services, including 409A valuations, Eqvista caters to the nuanced needs of private equity, ensuring that valuations are accurate and reflective of the current market conditions. Call us now to get started!